
OK, if the wind is blowing 10 mph from 9 o'clock and if my horizontal wind deflection is .7 inches at 100 yards, what is the drift at 1000 yards?
Well, roughly speaking, since the target is ten times more distant, the wind-drift must be around 7 inches, maybe a little more since the bullet will be slowing down. Seems reasonable right?
WRONG.
Lateral deflection by a 10mph crosswind is (roughly speaking) a function of the square of the distance. In this example, the TRUE deflection is .7" times (10x10) -- about 70 inches!!
I was going through some back issues of
Precision Shooting Magazine and this was explained in detail. Now, I remained skeptical, so I checked my
Pejsa Ballistics Calculator, which is just about the most accurate program I've found to describe the behavior of high BC bullets in flight.
For a 168 Sierra MK (.308), leaving the muzzle at 2700fps with a .464 BC, the numbers are as follows, with a 10mph, 9 o'clock crosswind:
Drift at 100: .75 moa (3/4")*
Drift at 200: 1.57moa (3.14")
Drift at 500: 5.06moa (25.3")
Quoting the article:
"Note that the deflections at 100 yards are typically a quarter of those at 200; lateral deflections increase as the square of the range". Precision Shooting, June 2000, p. 16.
For my 260 Rem load, 123 Scenar (.547BC), 2930fps, same 10mph conditions, Pejsa predicts:
Drift at 100: .48 moa (1/2")
Drift at 500: 2.73 moa (13.65")
Drift at 1000: 6.48 moa (64.8")
Again, even with a higher BC bullet, at 1000yds we end up with something pretty close to the 100-yard deflection (i.e. 1/2") multiplied by (10x10). Our calculated drift at 1000 is 64.8" -- that's over 100 times the 100-yard lateral drift in inches, even though the distance has only increased 10x.
* for simplicity, I'm calculating 1 moa = 1" x distance / 100 .
- - -
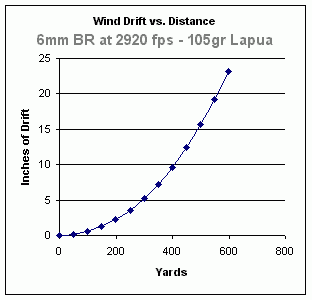
So, two things are apparent. One, you better have your windage on your come-up cards, and two, shoot fast or hope the wind doesn't change.
Obviously, if you set your 1000-yard 10mph windage based on what worked at 100, 200, or 300 yards you'll be way off. And, if there is a slight change in crosswind velocity, you won't even hit the target at 1000 yards.
The fact that lateral deflection increases with the SQUARE of the distance (roughly speaking) means that shooting at distance is ALL about gauging the wind -- and ranging your target precisely. And that's why it's WAY harder to shoot .5 moa at 1000 yards than it is to shoot .25 moa at 100 yards.- - -
Now I'm sure that many of the folks reading this are compensating correctly already, because they are shooting by the tables. But it is worth understanding the basic principle so that wind becomes less of a guessing game. The good news is that if you have correct windage calculations for 10mph, the corresponding numbers for a 5 mph wind are exactly half--at least THAT is an simple arithmetic ratio. For example.
123 Scenar, 2937fps Muzzle Velocity
Drift at 1000 (10mph): 6.48 moa (64.8")
Drift at 1000 (5mph): 3.24 moa (32.4")
and...
Drift at 100 (5mph): .24 moa (1/4")

But note even with a 5mph sidewind, the "Rule of the Square" still applies. The 1000-yard lateral deflection in inches is still over 100 times the lateral deflection at 100 yards, and you still have to dial in more than 10 times the windage moa.
But I've seen many shooters who go to the range, dial in windage at 100 or 200 yards, then don't understand why they can't hit anything at 500 yards even though the wind hasn't changed velocity. They need to reset their windage correction big time the further they go out.
Practical application -- wind reading vs. bughole accuracy.
The clear conclusion from the examples above is that for true long distance shooting, ones ability to assess actual cross-wind speed (and angle) over the course of bullet flight is VASTLY more important than whether ones rifle can shoot .25 moa or 1 moa.
To wit: Reducing accuracy from .25 moa to 1 moa puts you 7.5 inches off target at 1000yds. Misjudging a 9 o'clock wind by 5 mph puts you off target by roughly THREE FEET!- - -
Now, some would say--who cares about this--as long as I have a ballistic table with me. Fair enough, but knowledge of this basic "Rule of the Square" enables a shooter to make an informed guess about necessary windage even without a comeup sheet, as long as he knows the distance AND can fire a sighter at 100 or 200 yards as a baseline. For example. if I see empirically that I need 1" correction at 100yds, then I know at 600 yards I need (6x6) or 36 total inches of drift correction, or 6 moa.
Using the Pejsa calcs from above, how close will that estimate get you? Well, for the 168 SMK, a 1" correction at 100 yards corresponds to a 13 mph crosswind. The actual correction required is 7.36 moa.
So using your "Rule of the Squares" estimate, you're 1.36 moa off at 600 yards, or 8 inches. That's still good enough for a hit on a silhouette! And you accomplished this with no other knowledge than seeing your bullet hitting 1" away from point of aim at 100 yards.
The observed actual correction at 100 yards also tells you how fast the wind is blowing if you study your tables ahead of time. It is worth memorizing the 100-yard lateral deflection at various wind speeds. Of course a very small amount of horizontal wind drift at 100 yards is hard to distinguish from "operator error". But at 200 yards, if you start with a solid zero, your wind-driven impact change should be obvious after two or three sighters. (Remember the wind drift at 200 yards will be FOUR times the drift at 100 yards.) This should give you a solid basis to calculate the actual wind speed.
And once you know how hard the wind is
really blowing, then you can take full advantage of your come-up sheets and handheld ballistic calculators.
RELATED LINKS
FREE Ballistics Software: Use the JBM online ballistics program or download Pejsa and PointBlank Software.
Wind Drift by Gerard Schultz. Excellent technical Article which dispells many misconceptions about bullet performance. Useful charts plotting effect of both BC and distance on wind drift.
Copyright © 2004, 6mmBR.com, All Rights Reserved. No reproduction without advanced permission in writing.